Today we are going to finish-up our discussion of why the Sun appears to move the way it does and begin to move away from a geocentric view of the Universe. We will also attempt to understand the phases of the Moon.
We are now ready to talk about the motion of the Sun in a more complex way, by referencing its behavior to that observed at different locations on the Earth. First let's talk about the Sun as seen from Las Cruces. In the winter the Sun is very low in the sky at noon. For example, the altitude or elevation angle of the Sun in Las Cruces at noon on the 21st of December is only 34o. On June 21st, however, the elevation angle of the Sun is 81o: it is almost straight overhead (i.e., near the "zenith", altitude= 90o). Let's move to the equator. If we lived there, we would find that the Sun was exactly overhead (at noon) on March 21st, and again on September 21st. Between September and March, the Sun would be South of the zenith, between March and September, the Sun would be North of zenith.
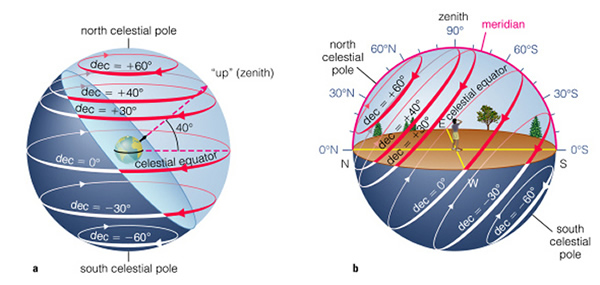
Let us now define zenith at the equator to be the "Celestial Equator". Objects to the north of the Celestial Equator are in the north celestial hemisphere, and objects to the south of the Equator are in the south celestial hemisphere. We can give this north-south direction a name, astronomers call it Declination. It is the "latitude" of the celestial sphere. In this system, Polaris has a Declination of +90o. The Celestial Equator has a Declination of 0o. Thus, an object is so many degrees north or south of the equator. Astronomers also have an equivalent to Longitude, it is called Right Ascension. Right Ascension ("RA") is measured in hours, and there are 24 hours in 360o. At the Celestial Equator, 1 hour = 15o. But like longitude, the angular size of an hour gets smaller as you head towards the Celestial Pole.
If you imagine a flat plane projecting out into space, the distance from one horizon to the other is 180o. Thus, you can see 180o of Declination, and 180o of RA, or 12 hours of RA at the celestial equator. An object on the Celestial Equator sets in the west 12 hours after it rose in the East. While an object at the North Celestial pole never rises or sets. Stars in between the Celestial Equator and the North Celestial pole are above the horizon for more than 12 hours (see Figure S1.15). Celestial objects that are South of the Celestial Equator are above the horizon for less than 12 hours. The geometry is shown in figure S1.15 of the textbook (on Page 100, reproduced here--note that this is for a latitude of 40o):

Now we are beginning to understand why the amount of daylight changes with season--the Sun appears to move above and below the celestial equator. On March 21st and September 21st, the Sun is on the Celestial Equator, and thus it is above the horizon for exactly 12 hours. On June 21st the Sun is at a Declination of 23.5 degrees North. In Las Cruces, this means that it is above the horizon for 14 hours. On December 21st, the Sun is at a Declination of 23.5 degrees South, so it is above the horizon for 10 hours each day. This is also the reason why the seasons are opposite to each other in the northern and summer hemispheres.
The length of daylight is one of the two controlling factors for why the weather is different in each of the seasons. If the Sun is up for a longer time each day, it can heat the air, soil and water for a longer time than if it was up for less time. Thus, there is more heating in summer than in winter. Note that in Las Cruces, the difference between the amount of daylight hours in the summer and winter is not very large (a factor of 1.4). That's why it is not as cold in Las Cruces as elsewhere during the winter. If you lived on the equator, the amount of daylight is (almost) the same every day during the year! Thus, it is always warm. But the opposite is true as you travel towards a pole. For example at a latitude of 57o there are only 6 hours of daylight during the winter. At latitudes above 66.5o, there are 0 hours of daylight on the 21st of December. In fact, at the North and South poles, there are six months each year when the Sun doesn't rise, and six months each year when the Sun is continually above the horizon! But even in summer it remains realtively cold at the poles, so the length of the day is not the only factor in determining the temperatures of the various seasons.
The other factor determining the nature of the seasons is the angle of the sunlight that hits the Earth. It is easily visualized in the drawing below:
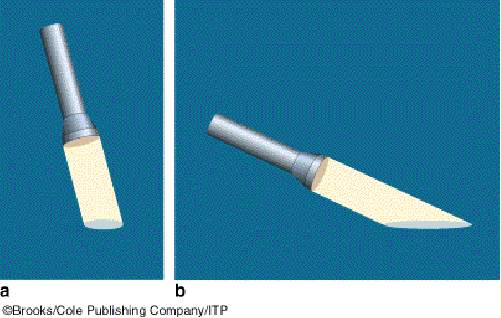
When the flashlight is nearly vertical (panel a), all of the light emitted by the flashlight is confined to a small area. If we tilt the flashlight (panel b), we see that the light is spread over a larger area. In Las Cruces, the Sun is nearly straight overhead during the summer, so the energy from the Sun is more concentrated. In the winter the intercepted energy from the Sun is spread over a larger angle because the Sun is lower down in our sky. At the poles, the Sun is always low down in the sky (it never gets higher than 23.5 degrees in elevation/altitude!). So the heating is much less efficient even though the Sun is up for 24 hours. This is how the seasons originate.
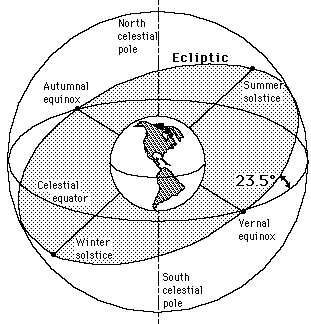
We have been considering the motion of celestial objects in a frame of reference where these objects circle the Earth--the geocentric model. But if you think about it, it is difficult to envision why the Sun and planets move the way they do. Careful observation shows that the Sun, Moon and Planets follow a specific path across the sky, through the same old constellations year after year. This path is called the "ecliptic", as this is where all solar and lunar eclipses occur. In the geocentric model, the spheres containing the Sun, Moon, and planets spin around an axis that is tilted with respect to the sphere containing the stars. The ecliptic is tilted by 23.5o to the celestial equator. "Damn, there's that 23.5o number again!".
We have to leave the geocentric view temporarily to understand why this number keeps cropping-up. In the next class we will see how the geocentric model was actually demolished. If we take a new view and say that the ecliptic defines a plane in space, that is the Sun, the Moon, and the planets can all be found on this plane, then this plane is tilted to the celestial equator, as shown in this figure:
Of course, what the ecliptic plane is actually about is that it is the plane in which the Earth orbits the Sun (all of the other planets orbit the Sun in almost the same exact plane). Here is a picture showing how the planets can all appear to be lined-up, which is the ecliptic plane (2.29):

As labeled on the diagram above, we now see the positions of the Sun on the ecliptic at the start of each of the four seasons (spring = Vernal Equinox). What is happening to cause this? The answer is that the Earth is spinning on its axis, and this axis remains tilted to its orbital plane, as shown in the figure below:

Remember that Polaris does not move--it stays fixed in the same position throughout the year. The Earth spins on its axis keeping this axis pointed at Polaris. This is demonstrated in this animation.
However, as the Earth goes around the Sun, this tilt causes a change in angle relative to the Celestial equator, and this is reponsible for the changing altitude of the Sun, and produces the seasons. For example, during the northern hemisphere summer (June 21st), the Earth's axis is pointed in a direction which makes the Sun appear to be higher in our sky than at other times during the year, as shown in this figure:

The unlabeled purple line in this figure is a latitude of +40o. The green line is the direction of the Sun, in this case it is the latitude on the Earth at which the Sun is straight overhead (at zenith) on June 21st, which is, of course, a latitude of +23.5o! [The "Tropic of Cancer".] In December, we have the opposite geometry:
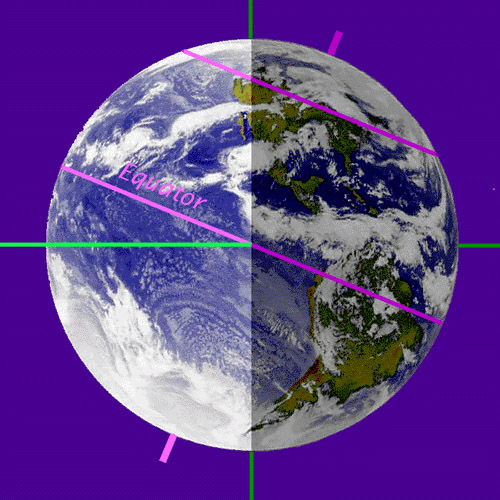
The Sun is now straight overhead for those people living at the southern latitude of -23.5o [the "Tropic of Capricorn"]. The Sun is now low in the sky for people in the northern hemisphere, but it is now summer in the southern hemisphere. It is also clear from these two diagrams what happens at the poles of the Earth. In the northern summer, you can see that the Sun never sets at the north pole, and in a small region near the north pole (see figure 2.17). In the northern winter, the Sun never rises above the horizon, so there are six months of darkness. The latitude defined by this effect is called the "arctic circle". What is the latitude of the arctic circle? Well, the north pole is at a latitude of +90o, and the Sun is 23.5o south of the celestial equator at the time of the Winter Solstice, so 90 - 23.5 = 66.5o. Here is an picture from our textbook (2.17) showing a summertime scene north of the arctic circle:

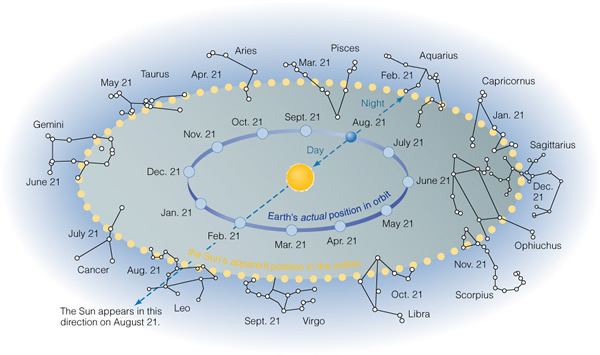
The sun makes an annual passage along the ecliptic, passing through the twelve zodiacal constellations (the 12 signs of the zodiac common to astrology), as shown in Figure 2.14:
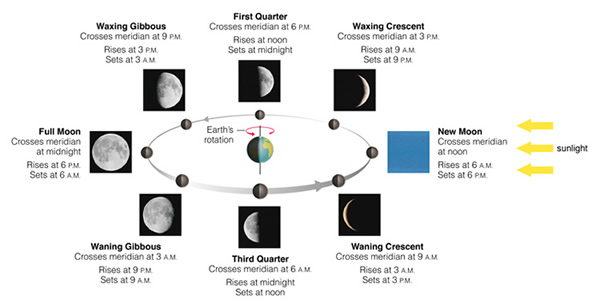
We have been exclusively talking about the motion of the Sun. Now lets understand the phases of the Moon. For the Moon, we can remain in a geocentric frame of mind because the Moon does go around the Earth! In fact, the ancient Greeks knew that the phases of the Moon were caused by the changing angle between the Sun, the Earth and the Moon--even though they believed that the Sun also orbited the Earth. Here is how the phases of the Moon change on a day-to-day basis over one cycle:

As we mentioned a few classes ago, the lunar cycle takes 29.5 days to go from full moon to full moon. In between there is the "New Moon" which we cannot see. Shortly after New Moon, we see a thin cresecent Moon in the west shortly after sunset (top left-most Moon image). As the days go on, this crescent becomes thicker, and thicker. About seven days after New Moon, the Moon appears as a half-circle (the seventh image in the sequence). This phase is called "first quarter". The Moon continues getting fuller, and fuller, until 14.75 days after New Moon, when "Full Moon" occurs. After full moon the disk shrinks back towards a half-illuminated phase ("last quarter"), and then continues to shrink into a crescent that rises shortly before the Sun in the eastern sky.
How can we explain these changes? As we already noted last class, the Moon has to be spherical--if it was a flat disk, then it could not assume any of the shapes shown above (except that of full Moon). Here is how the phases of the Moon are produced (similar to that from our textbook, Figure 2.21 on page 42):
As the Moon orbits the Earth, it presents an ever-changing phase as we see different portions of the illuminated hemisphere of the Moon. To make it clearer, let us change our perspective. If we were sitting high above the solar system, and looking down on the Earth-Moon system, we would see how the phases change with respect to the Sun-Earth-Moon angle:
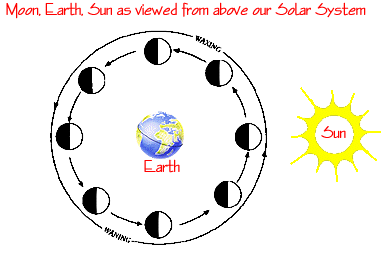
The Sun always illuminates exactly one half of the Moon (as it does the Earth). But, as the angle changes due to the Moon's motion in its orbit around the Earth, we see that the illuminated hemisphere of the Moon changes. At New Moon, the Moon is on a line between the Earth and the Sun, so the illuminated hemisphere cannot be seen--only the "dark side" of the Moon is pointed towards us. Because no part of the Moon (from our point of view) is illuminated, and the position of the Moon is close to that of the Sun, we cannot see the Moon when it is New. It takes about two days for the Moon to move far enough from the direction of the Sun to allow us to see the thin crescent in the western sky after sunset.
At first quarter, the angle between the Sun, Earth and Moon is 90o, and we see a half-moon. At full Moon, the angle between the Sun, Earth and Moon is 180o, and we see a fully illuminated hemisphere (Moon phase applet). Ok, now switching back to the view from Earth, we have the phases of the Moon (exactly corresponding to the positions of the previous figure):

If you look at the large set of 23 images of the phases of the Moon displayed previously, you will notice that the same features (on the Moon) are visible all of the time (at least when illuminated). For example, that roughly circular dark patch in the upper right hand quadrant of the Moon is the "Sea of Crises" (Mare Crisium). From Earth, only one side of the Moon is visible. There is a "near-side" (the side we see), and a "far-side" (the side we cannot see). Due to the gravitational pull of the Earth, the Moon rotates in a "synchronous" fashion. What this means is that the Moon spins on its axis exactly one time every orbit:
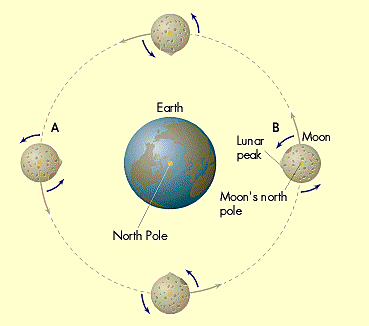
Many students get confused about the geometry of the orbit of the Moon because they have heard this term "the dark side" of the Moon (besides being a famous---at least to us old timers---album by Pink Floyd). If you examine the figures above about how the phases of the Moon change, you see that for at least one half of the lunar orbit, each spot on the Moon has daylight. That is there are 14.75 days when the Sun is visible each orbit, and 14.75 days each orbit when the Sun is not visible. At any one time (just like on the Earth!!!), there is one half of the Moon which is in darkness, and one half which is in the sunlight. It is true that the Moon spins much more slowly than the Earth, but the Moon has "days" and "nights". It is just that these days and nights are 14.75 Earth days long! So the far-side of the Moon which we cannot see is not the "dark side", it is the far-side. At full Moon, the far-side is completely dark. But at New Moon, the far-side is fully illuminated (as seen from the Sun!).
Some Ast 110 students are surprised when the look up and notice that they can see the Moon during the daytime. But this should not be a big surprise. Remember, from horizon to horizon is an angle of 180o. At first quarter Moon, the Moon, Earth and Sun make a 90o angle, thus, the Moon is separated by an angle of 90o from the Sun. If the Sun is setting on the Western horizon, then an angle of 90o means the Moon will be on the Meridian. A first quarter the Moon rises at Noon, crosses the Meridian at sunset, and sets in the West at midnight. But a full Moon is separated by 180o from the Sun--that is, exactly opposite to the Sun in the sky, so the full Moon rises at sunset, is on the Meridian at Midnight, and sets at sunrise!
Before we finish up with this subject, I just want to mention one more aspect of observing the Moon, and this is the phenomenon of Earthshine. When the Moon is a thin crescent (either waxing or waning), sometimes you can see features on the portion of the Moon that is not receiving direct sunlight. This phenomenon is shown in this picture of the crescent Moon:

This phenomenon is called "Earthshine". What is happening is that from the Moon, the Earth is almost "Full", and thus very bright to people standing on the Moon (because the Earth has clouds, the Earth reflects about 8 times more light than the Moon does). This light from the Earth can illuminate the dark hemisphere of the Moon, and cause it to softly glow. This aspect is best seen within a few days of New Moon.